ripple tank Spring 2004

A large-size ripple tank, 48 in x 30 in with water depth up to 2 in, was set up to study linear wave phenomena.

The main purpose of the project was to analyze and demonstrate diffraction - loosely, the ability of a wave to `slightly bend around a corner' or other sharp edge in its path, or - for light - to blur the light-dark boundary at the edge of a shadow.

Diffraction can occur in different types of linear waves, but the ripple tank uses waves or ripples on the surface of water below air to demonstrate it. First, we wanted to understand the behavior of this particular type of wave, and began by studying the equations that govern the motion of a fluid, and the conditions that hold at a water-air interface. From this, we found the `dispersion relation' that must be satisfied by time-harmonic, linearized waves propagating on the surface of water, which gives their speed as a function of their wavelength and the water depth.

This introduced the distinction between `dispersive waves', such as those at the water-air interface of the ripple tank, and `non-dispersive' waves, such as those governed by the classical wave equation of, for example, acoustics. Non-dispersive waves have a propagation speed that is independent of their wavelength, while for dispersive waves speed depends on wavelength. We also found out how, by selecting the water depth appropriately, the ripple tank could be operated in such a way that dispersion of waves was minimal. So, in our studies of diffraction, while the analysis was often based on the classical wave equation - because of its relative mathematical simplicity, and with its non-dispersive waves - we used the ripple tank (with its dispersive waves) to observe the effect experimentally.

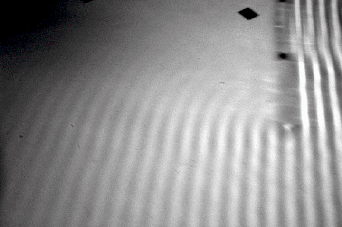
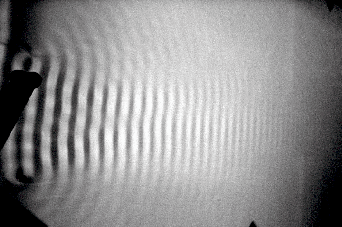
The distinction between dispersive and non-dispersive waves turned out not to matter for our studies of diffraction - because our old-and-borrowed wave generator could reliably produce a good, single-frequency (or `monochromatic') harmonic wavetrain. Here is an example, below. The dark corner in the foreground is showing that the point light source we used would not illuminate the whole area of the tank well - if we moved the light, we saw waves there.

  • We looked at the `Sommerfeld problem' for diffraction from the edge of a half-plane. The picture below shows the effect experimentally using the ripple tank, with the half-plane barrier at top-right.

  • Below are two views showing diffraction by a single slit or aperture of width about 7 wavelengths. We used approximate analytical methods to describe `Fresnel' diffraction, which occurs around the light-dark boundary or beam-edge near the source, and `Fraunhofer' diffraction, which occurs far from the source. The diffraction fringes of theory can be seen in these images fairly well.

  • To understand dispersion, we used the dispersion relation to compute water surface profiles at a sequence of times after the release of an initial square-shaped hump of water. (See, for example: Mathematics Applied to Continuum Mechanics, by Lee Segel. Dover Publications, 1987, Chapter 9.) The sequence of profiles (below) shows ripples propagating away from the initial disturbance and `breaking up' or dispersing into longer waves that travel faster, and therefore appear at the front of the disturbance, and shorter waves that travel more slowly and stay at the back.

  • We tried to demonstrate this using the ripple tank, with the initial disturbance made by pressing a ruler down into the water. The results after a few attempts were mixed - but perhaps if you download the movie and watch it slowed down, you will see longer waves passing through or overtaking shorter waves as they travel from the right to the left of the picture.

    • Movie: Ripple Tank Demonstration of Dispersion.